Understanding Einstein’s Theory

Einstein’s theories of relativity revolutionized our understanding of the universe. They are deeply connected to the concept of dimensions, particularly space and time. Here’s a breakdown of the theory in both contexts:

---

Einstein’s Theory Without Time-Space Dimensions

1. Key Idea: Energy-Mass Relationship
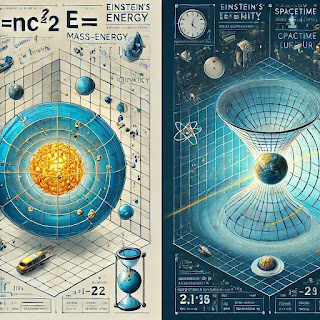
   Einstein's famous equation, $E = mc^2$, shows that energy ($E$) and mass ($m$) are interchangeable, connected by the speed of light squared ($c^2$).

   • This equation explains phenomena like nuclear energy, where small amounts of mass are converted into vast amounts of energy.

2. Implications:

   • It does not explicitly involve spatial or temporal dimensions but describes how mass and energy interact universally.
   • Foundation for understanding processes like nuclear reactions and star lifecycles.

---

Einstein’s Theory with Time-Space Dimensions

1. Special Relativity (1905):

   • Space and time are not separate entities but a single continuum called spacetime.
   • Time dilation: Moving objects experience time slower compared to stationary ones.
     • Example: Astronauts traveling near the speed of light age slower than people on Earth.
   • Length contraction: Objects in motion contract in the direction of movement as they approach the speed of light.

   Key Formula:

   $$t' = \frac{t}{\sqrt{1 - \frac{v^2}{c^2}}}$$

   Where $t'$ is the dilated time, $t$ is the stationary time, $v$ is the velocity, and $c$ is the speed of light.

2. General Relativity (1915):

   • Gravity is not a force but the warping of spacetime by massive objects.
   • Objects follow the curved paths in spacetime, like planets orbiting stars.
   • Predicts phenomena like black holes and gravitational waves.

   Key Equation: Einstein’s field equations relate spacetime curvature ($G$) to energy and matter ($T$):

   $$G_{\mu\nu} + \Lambda g_{\mu\nu} = \frac{8\pi G}{c^4} T_{\mu\nu}$$

---

Illustrative Examples

1. Without Dimensions:
   Imagine mass-energy conversion in a nuclear reactor—no explicit reference to time or space is required to understand the transformation.

2. With Dimensions:
   The bending of light near a massive object (gravitational lensing) demonstrates spacetime warping. GPS systems correct for time dilation caused by Earth’s gravity, showcasing relativity's practical application.

---

Impact of Einstein’s Theories

• Technology: GPS, nuclear energy, and advanced space exploration.
• Philosophy: A deeper understanding of time and space reshaped human perception of reality.
• Astronomy: Explains the behavior of black holes, expansion of the universe, and cosmic phenomena.

---

Here is an educational illustration of Einstein's theory of relativity, demonstrating both mass-energy equivalence and spacetime curvature. Let me know if additional elements or adjustments are needed!